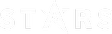Practical Exercise 2: A SIMPLE KEPLER-TYPE TELESCOPE

A simple Kepler-type telescope can be constructed from a pair of magnifying glasses. Larger commonly sold magnifying glasses (diameter 9 - 10 cm) have focal length of about 40 cm, medium ones (diameter 5 - 6 cm) about 15 cm and the smallest ones have focal length of about 10 cm (see Fig. 4). To construct a telescope, we need one small magnifying glass and one medium one, or rather a large one.

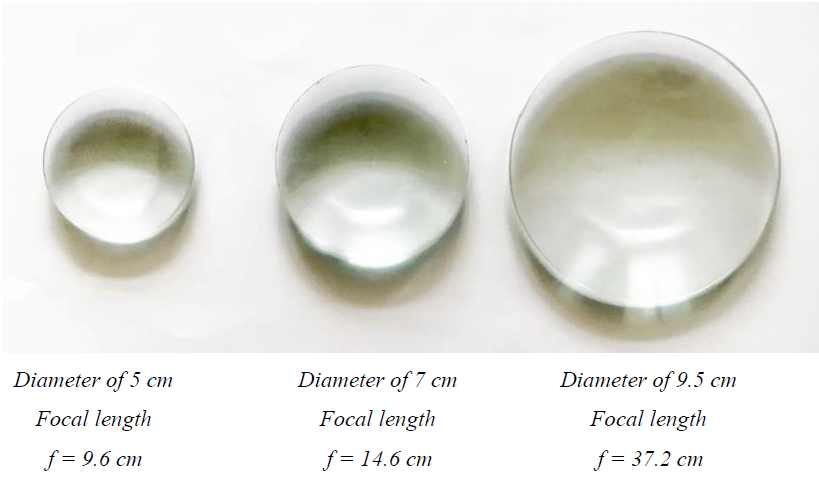
Figure 4: Available converging lenses (magnifying glasses)

1. Determine the focal length of the lenses used. The easiest way to do this is to place the lens (use e. g. a laboratory stand, or just a hand when there is no other option) under a source of light and try to create a sharp image of the source on the table or floor above the lens. The height of the lens above the image (table, floor) equals to the focal length of the lens. Write the measured values in the table:

Measuring No.	f_ob/cm
1	37,3
2	36,8
3	37,5
4	37,2
5	32,2

f_ob = 37,2 cm

Measuring No.	f_ok/cm
1	9,5
2	9,6
3	9,4
4	9,7
5	9,9

f_ok = 9,6 cm

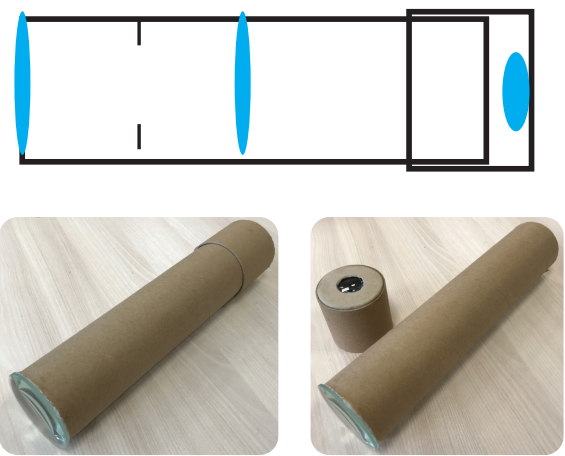
2. Place the objective lens and ocular lens in a suitable tube. A perfect choice is a paper case for drawings, while the ocular lens can be attached to the stopper that closes the tube. The telescope can be focused by moving the stopper at the end of the tube.

Figure 5: Scheme of Kepler telescope and a photograph of its realization

Never look at the sun through the telescope! This could cause an irreversible damage to the eyes!

3. With your telescope you can observe terrestrial objects, and you can quickly make sure that the image is indeed inverted laterally and vertically. For instance, when observing the Moon in its full phase, you will also find out that light is actually dispersed.

Sample worksheet solution:

Length of the telescope = f_ob + f_ok = 37,2 cm + 9,6 cm = 46,8 cm

Magnification of the telescope

In practice, in astronomical telescopes, parameters are often given in the format

diameter of the objective lens / focal length of the objective lens.

Finally, write down the parameters of the telescope you constructed: 90/372 mm.

If the ocular lens from the telescope is available, a direct comparison can be made. When observing a square grid it is clear that distortion in professional ocular lenses is much better compensated:

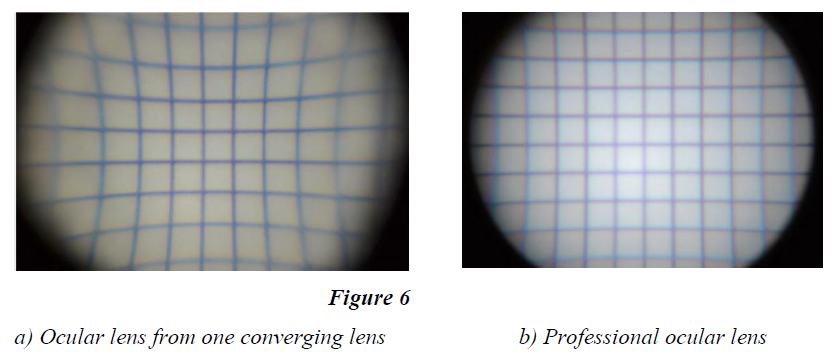
Figure 6

Additional Materials

Worksheet 2