- Practical exercise 1 AROUND THE SUN
- Practical exercise 2 THE MOON IN THE DISTANCE…HOW FAR??
- Practical exercise 3 THE NEIGHBOR ALSO LIKES IT
- Practical exercise 4 ALIEN VS. EARTHLING
- Practical exercise 5 GAS GIANT JUPITER
- Practical exercise 6 MARS! ...WHERE?
- Practical exercise 7 FLIGHT TO MARS ALONG HALF ELLIPSE
- Practical exercise 8 GEOSTATIONARY SATELLITE
- Practical exercise 9 CATASTROPHE ON THE SUN
- Practical exercise 10 LIGHT AS... SUPERMASSIVE BLACKHOLE!
Practical exercise 7: FLIGHT TO MARS ALONG HALF ELLIPSE
A spaceship has taken off from Earth, moving along an elliptical orbit with the Sun in focus. The flight parameters are set so that the Earth (the place from which the ship took off) is the perihelion and the ship reaches a point exactly on the opposite side of the ellipse, i.e. the aphelion where Mars will be located. The ship therefore moves along a half ellipse (the so called Hohmann transfer orbit). The semi major axis of the Earth's trajectory is and of Mars
.
Determine:
a) the semi major axis of the ellipse,
b) how much time will the crew have to spend in the spaceship before the ship reaches Mars.
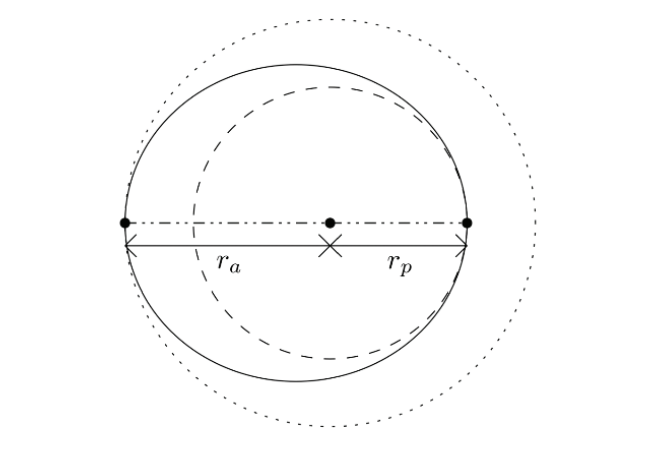
Fig. 6 The picture shows three points (from left to right): Mars, Sun, and Earth
Riešenie:
a) It is clear from the figure that , i.e.
.
b) We determine the orbital period from the 3rd Kepler's law: , so the period is T = 1,42 rokov. The spaceship moves along the half of the ellipse, so the time needed to reach Mars is half the period: 0.71 years, i.e. 8.5 months